Sequence and series are closely related concepts and possess immense importance. Those sequences whose terms follow certain patterns are called progressions. Progressions (or Sequences and Series) are numbers arranged in a particular order such that they form a predictable order. By predictable order, we mean that given some numbers, we can find next numbers in the series.
Progression is of three types:
1. Arithmetic Progression.
2. Geometric Progression.
3. Harmonic Progression.
Arithmetic Progression
It is a sequence of numbers in which each subsequent term of it is obtained by adding up a constant term to it. That is, the difference between two consecutive terms remains same throughout. This constant term is known as Common Difference and is denoted by ‘d’ and the first term of the sequence is denoted by ‘a’. This progression is of form a, a+d, a+2d, a- +3d… For instance, consider the sequence 2, 5, 8, 11, 14, 17. You can notice that in this example the difference between any two terms of the sequence is 3 (5-2=3, 8-5=3, 11-8=3 and so on.) Therefore, in this AP the common difference ‘d’ is 3 and first term ‘a’ is 2.
Example 1
The first term of A.P. is 1 and the common difference is 5 which calculates the 7th term of this sequence.
Solution
The sequence can be formed as 1, 6, 11, 16… and we are interested to get the 7th term of this sequence. We can write it in the form a + 6d and thus get 31. Generalizing it, we get a + (n1)d. Hence, the formula we get for the nth term of A.P. is denoted by
Tn = a+ (n-1)d.
What if you have to calculate the sum to n in terms of the above sequence then you can use the formula is given as
Sn = n/2 {2a + (n-1) d}
or
Sn = n/2 {a + l},
where l is the last term of A.P.
Thus, using this formula we get S7 = 112.
Example 2
The sum of the first three no. of A.P. is 27 and the sum of their squares is 293. Then find the numbers.
Solution
Assume the three terms to be a-d, a, a+d. Since, the sum of first three terms is 27. So,
a-d + a+ a + d = 27
Canceling out d we get,
3a = 27
a = 9
Also, (a-d)² + a² + (a+d)² = 293
243 + 2d² = 293
2d² = 50
d² = 25
d = ±5
When d= -5, the no.’s is 14, 9, 4
When d= 5, the no’s are 4, 9, and 14.
Thus, by using this approach we can quickly and easily determine the three numbers.
Likewise, if terms are more than 3 then follow up the table given below.
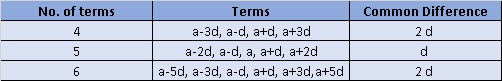
Relationship between Arithmetic Mean and A.P.
Let A be the arithmetic mean of a and b. Then, a, A, b are in A.P.
Therefore A – a = b – A => 2A = a + b = (a + b)/ 2
Geometric Progression:
This is a kind of sequence where each term after the first is obtained by multiplying the previous no. by a fixed constant number, known as the common ratio. It is denoted by ‘r’. It is
of form a, ar, ar², ar³…
The nth term of the G.P. is
Tn = ar(n-1)
For example, 2, 4, 8, 16, 32…so on is a G.P. with common ratio = ak+1/ak = 2.To calculate the sum to n terms of G.P. of the above example use this formula.
Sn = a(1-rn/ 1-r) = a/1-r -arn/1-r
And when n →∞ then rn →0
Thus, some of an infinite G.P. is given by
S = a/1-r
Where r is a common ratio and a is the first term of G.P.
Suppose, 1/23 , 1/26, 1/29…is an infinite G.P. Then its sum will be
S = 1/23/ (1 – 1/8) = 1/7
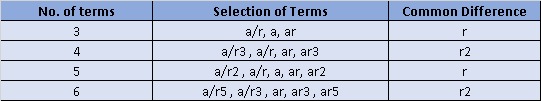
As given in the above example of A.P. in case of selection of terms similar kinds of questions can also be asked for G.P. where you have been provided the sum of the first 3 or 4 terms of G.P. and you need to find out the numbers. You can refer to the table given below for the same.
Question
The sum of the first two terms of G.P. is 2 and the sum of the first four terms of G.P is 20. Find the G.P.
Solution
Here we won’t take terms of G.P. to be a/r3, a/r, ar, ar3 as this question is not based on a selection of terms
In this question, we will take the terms a, ar, ar2, and ar3.
Since, a + ar = 2 => a (1 + r) = 2
Also, a+ ar +ar2+ ar3 = 20 => a (1 + r) (1 + r2) = 20
⇒2(1 + r2) = 20
⇒(1 + r2) = 10
⇒r2 = 9
⇒r = ±3
⇒a = -1, when r = -3
⇒a = ½, when r = 3.
Relationship between Geometric Mean and G.P.:
Let G be the geometric mean between a and b. T
hen a, G, b are in G.P. And, G = √ab.
Harmonic Progression
It is a progression that is formed by taking the reciprocal of the arithmetic mean. It is of the form 1/a, 1/(a+d), 1/(a+2d) … For example, 1, 3, 5, 7, 9 forms an A.P. with common difference = 2 then H.P. will be 1, 1/3, 1/5, 1/7, and 1/9. If the sum of the first 2 terms of H.P. is 17/20, the sum of the next two terms of H.P. is 5/4, and the sum
of the following two terms is -7/10. Find the sum of the following two terms again.
In this question, we are given
1/a + 1/(a+d) = 17/20
1/(a+2d) + 1/(a+3d) = 5/4
1/(a+4d) + 1/(a+5d) = -7/10
Solving all 3 equations simultaneously we get, a = 10, d = -3.
Therefore, 1/(a+6d) +1/(a+7d) = -19/88.
Relationship between Harmonic Mean and H.P.
Let H be the harmonic mean between two non-zero numbers. Then a, H, and b are in H.P. where
H = 2ab/(a+b). So, 1/a, 1/H, and 1/b are in A.P.
Question
The sum of three numbers in a GP is 26 and their product is 216. Find the numbers.
Solution :
Let the numbers be a/r, a, ar.
=> (a / r) + a + a r = 26
=> a (1 + r + r2 ) / r = 26
Also, it is given that product = 216
=> (a / r) x (a) x (a r) = 216
=> a3 = 216
=> a = 6
=> 6 (1 + r + r2) / r = 26
=> (1 + r + r2) / r = 26 / 6 = 13 / 3
=> 3 + 3 r + 3 r2 = 13 r
=> 3 r2 – 10 r + 3 = 0
=> (r – 3) (r – (1 / 3) ) = 0
=> r = 3 or r = 1 / 3
Thus, the required numbers are 2, 6, and 18.
Question
Find the sum of all the terms, If the first 3 terms among 4 positive 2-digit integers are in A.P and the last 3 terms are in G.P. Moreover the difference between the first and last term is 40.
Solution
Let the numbers be x-a, x, x+a
∴ For the last 3 terms to be in G.P:- (x+a)2 = x(x-a+40) x
2+a2+2ax = x2 -ax+40x a
2 = -3ax + 40x
=> x = a2(40−3a)a2(40−3a)
Now, since x is a positive integer, putting the different integral values of a.
Only a = 12 gives a integer value of x = 36
(Note, a=b also give integral value x=b but then the first term becomes 0)
∴ x = 36, a = 12
∴ x – a = 24
x = 36
x+a = 48
x-a+40 = 64
∴ Sum of all the terms = 64+48+36+24 = 172
The terms are – 24,36,48,64
Answer 172
