CAT Geometric Progression is an important topic for preparations. Geometric Progression is most interesting concepts of mathematics. The geometric progression is a sub-topic of progression and series. Aspirants should expect a few questions from Geometric Progression. As quantitative section contain a total of 34 questions. Out of that few questions are from Geometric Progression in CAT exams.. A Geometric Progression is contains both theory and practical questions.

Clearing your Geometric Progression concepts will enhance your accuracy level in exams. Hence, Pay attention to concepts and formulas. As you all know Geometric Progression topics contain good weightage in the CAT exam. That's why this become an important topic for exams.

In this article, we learn about some of the important basic rights of CAT Geometric Progression. All important aspects related to the topic will be covered. The aspirant would understand how to approach questions when his concepts become clear. In addition, one could easily ace Geometric Progression just by formulas and basic concepts clarity.

Also read: How to Prepare for CAT 2022

Meaning of Geometric Progression in CAT exam.

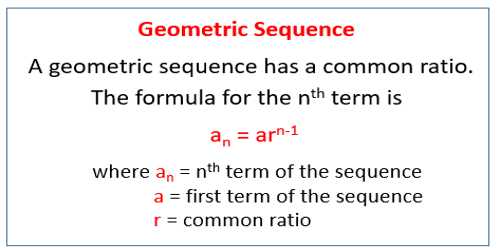
A geometric progression or a geometric sequence is the sequence, in which each term is varied by another by a common ratio. The next term of the sequence is produced. When we multiply a constant (which is non-zero) to the preceding term. It is denoted as:

a, ar, ar², ar³, ar⁴, and so on.

Where a is the first term and r is the common ratio.

Note: It is to be noted that when we divide any succeeding term from its preceding term. Then we get the value equal to the common ratio.

Suppose we divide the 3rd term by the 2nd term we get: ar²/ar = r

In the same way: ar³/ar² = r; ar⁴/ar³ = r

Properties of Geometric Progression in CAT exam.

Properties of geometric progression are mentioned below:

1. Three non-zero terms a, b, c are in GP if and only if b² = ac
2. In a GP,
   Three consecutive terms is show as a/r, a, ar
   Four consecutive terms is show as a/r³, a/r, ar, ar³
   Five consecutive terms can be taken as a/r², a/r, a, ar, ar²
3. In a finite GP, the product of the terms equidistant from the beginning and the end is the same
   That means, t₁.t_n = t₂.t_n-1 = t₃.t_n-2 = …..
4. If each term of a GP is multiplied or divided by a non-zero constant, then the resulting sequence is also a GP with the same common ratio
5. The product and quotient of two GP’s is again a GP
6. If each term of a GP is raised to the power by the same non-zero quantity, the resultant sequence is also a GP
7. If a₁, a₂, a₃,… is a GP of positive terms then log a₁, log a₂, log a₃,… is an AP (arithmetic progression) and vice versa

Common Ratio of GP

Consider the sequence a, ar, ar², ar³,……

First term = a

Second term = ar

Third term = ar²

Similarly, nth term, t_n = ar^n-1

Thus, the common ratio of geometric progression formula is show as:

Common ratio = (Any term) / (Preceding term)

t_n / t_n-1

(ar^{n – 1} ) /(ar^{n – 2}) = r

Thus, the general term of a GP is show as ar^n-1 and the general form of a GP is a, ar, ar²,…..

For Example: r = t₂ / t₁ = ar / a = r

Types of Geometric Progression in CAT exam.

Geometric progression is divide into two types based on the number of terms it has. They are:

• Finite geometric progression (Finite GP)
• Infinite geometric progression (Infinite GP)

These two GPs are explained below with their representations and the formulas to find the sum.

Finite Geometric Progression

The terms of a finite G.P. is show as a, ar, ar², ar³,……ar^n-1

a, ar, ar², ar³,……ar^n-1 is called finite geometric series.

The sum of finite Geometric series is:

Infinite Geometric Progression

Terms of an infinite G.P. is show as a, ar, ar², ar³, ……ar^n-1,…….

a, ar, ar², ar³, ……ar^n-1,……. is called infinite geometric series.

The sum of infinite geometric series is: ∑k=0∞(ark)=a(11−r)

So, The geometric progression formula of sum to infinity.

Tricks for Geometric Progression

Follow these strategies to solve many sorts of geometrical progression mathematics problems quickly:

1. Consider the integers ar,a,ar, where r is the common ratio. Where the product of three geometric progression terms.

2. Consider the integers ar3,ara,ar3. When the product of four geometric progression terms. Where r is the common ratio.

3. Consider the integers ar2 ,ara,ar,ar2, where r is the common ratio. When the product of five terms of the geometric progression.

Formula of Geometric Progression

Each term in a geometric progression is obtain. If multiplying the previous term by a set number

(common ratio).

In general, the progressions is show as follows:

a, ar, ar2, ar3,……

Except for the first term, each term is derived by multiplying the previous term by the common ratio.

a-First-term

r-Common ratio

Video's that could help aspirant.

Solved Examples of G.P

For Example 1: If the first term is 10 and the common ratio of a GP is 3, then write the first five terms of GP.

Solution: Given, First term, a = 10

Common ratio, r = 3

We know the general form of GP for first five terms is show as:

a, ar, ar², ar³, ar⁴

a = 10

= ar = 10 × 3 = 30

ar² = 10 × 3² = 10 × 9 = 90

= ar³ = 10 × 3³ = 270

ar⁴ = 10 × 3⁴ = 810

Therefore, the first five terms of GP with 10 as the first term and 3 as the common ratio are: 10, 30, 90, 270 and 810

For example 2: Find the sum of GP: 10, 30, 90, 270 and 810, using formula.

Solution: Given GP is 10, 30, 90, 270 and 810

First term, a = 10

Common ratio, r = 30/10 = 3 > 1

Number of terms, n = 5; Sum of GP is show as;

S_n = a[(rⁿ – 1)/(r – 1)]

S₅ = 10[(3⁵ – 1)/(3 – 1)]

= 10[(243 – 1)/2]

so, 10[242/2] = 10 × 121 is equal to 1210

Check: 10 + 30 + 90 + 270 + 810 = 1210

For example 3: If 2, 4, 8,…., is the GP, then find its 10th term.

Solution: The nth term of GP is show as: 2, 4, 8,….

Here, a = 2 and r = 4/2 = 2

a_n = ar^n-1

Therefore, a₁₀ = 2 x 2^{10 – 1} = 2 × 2⁹ = 1024

Practice Problems on Geometric Progression

1. Find the equivalent fraction of the recurring decimal 0.595959…..
2. What is the 12th term of the sequence 4, -8, 16, -64,….?
3. Check whether the given sequence is GP.
   27, 9, 3, …
4. Write the first five terms of a GP whose first term is 3 and the common ratio is 2.

For CAT Quantitative section’s full guidance visit this link- https://catking.in/best-guide-for-preparation-of-cat-quantitative-ability/

ENROL ON OUR COURSES NOW!

For CAT courses AND For Non-cat courses