The CAT: Quadrilateral basic concepts.
CAT Quadrilateral is an important topic for preparations. It is part of geometry questions. As geometry contains 10% weightage in CAT exams. Aspirants should expect at least 5-6 questions from Quadrilateral. As quantitative section contain a total of 34 questions and out of that 5-6 are Quadrilateral problems. A quadrilateral is the most formula and fact-based topic.
Clearing your Quadrilateral concepts will enhance your accuracy level in exams. Hence, Pay attention to concepts and formulas. As you all know geometry topics contain 10% of the weightage in the CAT exam.
In this article, we learn about some of the important dimensions of CAT Quadrilateral. All important aspects related to the topic will be covered. The aspirant would understand how to approach questions when his concepts become clear. In addition, one could easily ace Quadrilateral just by formulas and basic concepts clarity.
Also read: How to Prepare for CAT
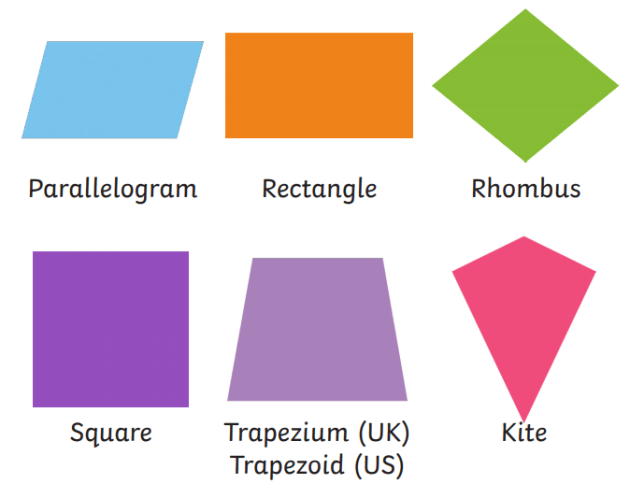
The basic concept of Quadrilateral for CAT exam.
A quadrilateral is a plane figure that has four sides or edges, and also four corners or vertices. The angles are present at the four vertices or corners of the quadrilateral. If ABCD is a quadrilateral then angles at the vertices are ∠A, ∠B, ∠C and ∠D. The sides of a quadrilateral are AB, BC, CD and DA.
If we join the opposite vertices of the quadrilateral, we get the diagonals. In the below figure AC and BD are the diagonals of quadrilateral ABCD. Quadrilaterals will typically be of standard shapes with four sides. For example rectangle, square, trapezoid, and kite or irregular and uncharacterized as shown below:
Therefore, Let’s have a look at the table below to understand quadrilaterals more clearly
| Quadrilateral | Two-dimensional plane figure enclosed by 4 line segments/ A polygon with 4 edges and 4 vertices |
| Number of sides | 4 sides |
| Number of vertices | 4 vertices |
| Number of diagonals | 2 diagonals |
| Sum of all interior angles | 360 degrees |
| Sum of all exterior angles | 360 degrees |
Types of Quadrilateral for CAT exam.
The types of quadrilaterals are defined based on the measure of the angles and lengths of their sides. As the word ‘Quad’ means four, all these types of quadrilateral have four sides. Therefore, the sum of angles of these shapes is 360 degrees. So have a look at the list of types of quadrilaterals are:
- Trapezium
- Parallelogram
- Squares
- Rectangle
- Rhombus
- Kite
Sides and Angles of Quadrilaterals
| Sides and angles | Square | Rectangle | Rhombus | Parallelogram | Trapezium |
| All sides are equal | Yes | No | Yes | No | No |
| Opposite sides are parallel | Yes | Yes | Yes | Yes | Yes |
| Opposite sides are equal | Yes | Yes | Yes | Yes | No |
| All the angles are of the same measure | Yes | Yes | No | No | No |
| Opposite angles are of equal measure | Yes | Yes | Yes | Yes | No |
| Diagonals bisect each other | Yes | Yes | Yes | Yes | No |
| Two adjacent angles are supplementary | Yes | Yes | Yes | Yes | No |
Quadrilateral Formulas for CAT exam.
There are two basic formulas for quadrilaterals, that are:
- Area
- Perimeter
Area of Quadrilateral
The area of the quadrilateral is the total space occupied by the figure. So the area formula for the different quadrilaterals is given be
| Parallelogram Area | Base x Height |
| Rectangle Area | Length x Width |
| Square Area | Side x Side |
| Rhombus Area | (1/2) x Diagonal 1 x Diagonal 2 |
| Area of a Kite Area | 1/2 x Diagonal 1 x Diagonal 2 |
Perimeter of Quadrilateral
Perimeter is the total distance covered by the boundary of a 2d shape. Since we know the quadrilateral has four sides, therefore, the perimeter of any quadrilateral will be equal to the sum of the length of all four sides. If ABCD is a quadrilateral then, the perimeter of ABCD is:
Perimeter = AB + BC + CD + AD
| Quadrilateral Name | Perimeter |
| Square | 4 x Side |
| Rectangle | 2(Length + Breadth) |
| Parallelogram | 2(Base + Side) |
| Rhombus | 4 x Side |
| Kite | 2 (a + b), a and b are adjacent pairs |
Convex, Concave and Intersecting Quadrilaterals
Another way to classify the types of quadrilaterals ais
- Convex Quadrilaterals: Both the diagonals of a quadrilateral are completely contained within a figure.
- Concave Quadrilaterals: At least one of the diagonals lies partly or entirely outside of the figure.
- Intersecting Quadrilaterals: Intersecting quadrilaterals are not simple quadrilaterals in which the pair of non-adjacent sides intersect. These kinds of quadrilaterals are known as self-intersecting or crossed quadrilaterals
Property of Quadrilateral for CAT exam.
| Rectangle Property | Rectangle have 4 right angles and its opposite sides are equal |
| Square Property | Square also have 4 right angles and it has 4 equal sides. |
| Parallelogram Property | Parallelograms have two pairs of parallel sides and opposite sides equal. |
| Rhombus Property | Rhombus is a parallelogram with 4 equal sides. |
| Trapezoid Property | Trapezoid have two sides parallel |
| Kite Property | Kite has two pairs of adjacent sides of the same length. |
Some sample questions with solutions.
For Example 1: What is the base of a rhombus, if its area is 40 square units and the height is 8 units?
Solution: Given, Area = 40 square units
Height = 8 units
Area of rhombus = Base × Height
40 = Base × 8
So, The Base = 40/8 = 5 units
For Example 2: If 15 metres and 6 metres are diagonal lengths of a kite, then what is its area?
Solution: Given, diagonal 1 = 15 metres and diagonal 2 = 6 metres. So, the area is simply calculated as, (1/2)(15×6) = 45 m2.
For Example 3: Find the perimeter of the quadrilateral with sides of 5 cm, 7 cm, 9 cm and 11 cm.
Solution: Give the n, sides of a quadrilateral 5 cm, 7 cm, 9 cm and 11 cm.
Therefore, the perimeter of a quadrilateral is:
Hence, P = 5 cm + 7 cm + 9 cm + 11 cm = 32 cm
For Example 4: The perimeter of the quadrilateral is 50 cm and the lengths of the three sides are 9 cm, 13 cm and 17 cm. Find the missing side of the quadrilateral.
Solution: Let the unknown side of the quadrilateral = x
Given, Perimeter of the quadrilateral = 50 cm
The lengths of the other three sides are 9 cm, 13 cm and 17 cm
As we know,
Perimeter = sum of all the four sides.
50 = 9 cm + 13 cm + 17 cm + x
50 = 39 + x
x = 50 – 39
x = 11, Therefore, the fourth side of the quadrilateral = 11 cm
Some questions for practice.
Question1: The rhombus of side 6 cm has an angle equal to the external angle of a regular octagon. Find the area of the rhombus.
Question2: The perimeter of the quadrilateral is 50 cm and the lengths of the three sides are 9 cm, 13 cm and 17 cm. Find the missing side of the quadrilateral.
Question3: In a trapezium ABCD, Calculate ∠C and ∠D if ∠A = 55° and ∠B = 70°
Question4: Find all the angles of a parallelogram if one angle is 80°.
Question5: In a rectangle, one diagonal is inclined to one of its sides at 25°. Measure the acute angle between the two diagonals.
For CAT Quantitative section's full guidance visit this link- https://catking.in/best-guide-for-preparation-of-cat-quantitative-ability/
ENROL ON OUR COURSES NOW!
